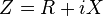Acoustic impedance
What is acoustic impedance?[edit | edit source]
A useful quantity in acoustics is impedance. It is a measure of the amount by which the motion induced by a pressure applied to a surface is impeded. Or in other words: a measure of the lumpiness of the surface. Since frictional forces are, by and large, proportional to velocity, a natural choice for this measure is the ratio between pressure and velocity. A quantity, however, that would vary with time, and depend on the initial values of the signal is not very interesting. Therefore, impedance is defined via the Fourier transformed signal as: Acoustic impedance (which has the symbol Z) is the ratio of acoustic pressure (p) to acoustic volume flow (U). So it's define like Z = p/U. Z usually varies strongly when you change the frequency. The acoustic impedance at a particular frequency indicates how much sound pressure is generated by a given air vibration at that frequency.
The specific acoustic impedance[edit | edit source]
The specific acoustic impedance z is a ratio of acoustic pressure to specific flow, which is the same as flow per unit area, or flow velocity. In all cases, 'acoustic' refers to the oscillating component. Acoustic impedance Z = pressure/flow and specific acoustic impedance z = pressure/velocity.
Units[edit | edit source]
The unit of pressure is the pascal – one newton per square metre. A pascal is a big unit for sound: an oscillation of one Pa is usually a very loud sound indeed. (In DC the Pa seems a small unit: atmospheric pressure is 100,000 Pa or 100 kPa.) Flow is measured in cubic metres per second. The units for impedance are therefore Pa.s/m3, which we call the acoustic ohm Ω. For musical instruments, it is a rather small unit, so we use megohms: MPa.s/m3. Sound pressures have a large range.
Complex impedance[edit | edit source]
In general, a phase relation exists between the pressure and the particle velocity. The complex impedance is defined as
where R is the resistive part, and X is the reactive part of the impedance The resistive part represents the various loss mechanisms an acoustic wave experiences such as random thermal motion. For the case of propagation through a duct, wall vibrations and viscous forces at the air/wall interface (boundary layer) can also have a significant effect, especially at high frequencies for the latter. For resistive effects, energy is removed from the wave and converted into other forms. This energy is said to be 'lost from the system'. The real part of the impedance (the resistance) is related to the energy flow: if Re(Z) > 0, the surface is passive and absorbs energy; if Re(Z) < 0, it is active and produces energy.
Links[edit | edit source]
Related articles[edit | edit source]
External links[edit | edit source]
Bibliography[edit | edit source]
http://en.wikipedia.org/wiki/Acoustic_impedance
"An Introduction to Acoustics" S.W. Rienstra & A. Hirschberg, Eindhoven University of Technology, - 9.12.2012