Resolution of an eye
Resolution of an eye
Introduction
Resolution (SPATIAL RESOLUTION) is the ability to see fine detail in an image; it can be quantified by separation of two distinguishable points. According to text books at this level the resolution an optical instrument is dependent on the size of the aperture; this is one of the reasons why high resolution telescopes have such large diameter mirrors. The eye is an optical instrument that has a small aperture, called pupil, according to the same principle its resolution should therefore be dependent on the pupil size which would imply that our eyes could resolve more detail in the dark when the pupil is large than in bright light when it is small, this seems strange so in this essay the question “Is the resolving power of the eye affected by the size of the pupil?” will be addressed.
Diffraction by small apertures
When light passes through a small aperture it spreads out to produce an intensity pattern shown in pic.1, this is called diffraction and can be explained using Huygens’ construction. This models the wave front as an infinite number of small wavelet sources each propagating a spherical wave in the forward direction, these wavelets can be summed to give the resultant intensity at any given angle. Using this model it can be shown that the angular separation of the first minima θ = 1.22λ/a where a is the diameter of the aperture. If an image of a point object is produced by an optical instrument diffraction at the aperture will cause the image to be a series of rings rather than a point.
/ Pic.1 Diffraction of light by a circular aperture
Pic.1 Diffraction of light by a circular aperture
Rayleigh Criterion
The Rayleigh criterion is a way of defining the SPATIAL RESOLUTION of an optical instrument, this states that two images are just resolvable when the centre of the diffraction disk of one is directly over the first minimum of the other.This is illustrated in pic.2. The outcome of this is that the SPATIAL RESOLUTION of an optical instrument is dependent on the size of the aperture.
<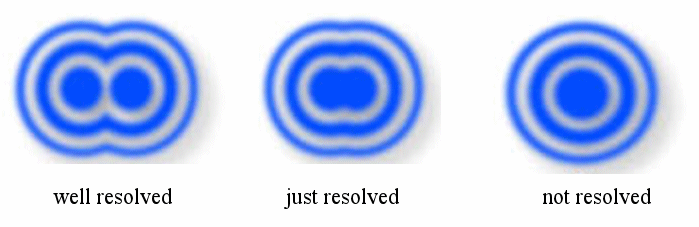 >
Pic. 2 Rayleigh Criterion
>
Pic. 2 Rayleigh Criterion
The Human Eye
Light enters the eye (Pic.3) through the pupil and is focused on the retina by the lens. The retina is made up of millions of light sensitive cells that convert the light to a nerve impulse that can be sent to the brain via the optic nerve. The cells of the retina do not work well if the light is either too bright or too dark; to optimise the amount of light entering the eye the iris controls the size of the pupil making it smaller when the light is bright and bigger when the light is dark. The average size of the pupil can vary from 3-9mm . Since the amount of light entering the eye is proportional to the diameter squared the pupil can vary the light by a factor of 9, this is not enough to deal with the difference from seeing in bright sunlight to seeing stars at night a factor 107 this is managed by chemical changes that take place in light sensitive cells themselves combined with using different cells for low light conditions.
< > Pic.3 Human eye
> Pic.3 Human eye
The SPATIAL RESOLUTION of the eye
The SPATIAL RESOLUTION can be quantified by the angle subtended between the two closest points that can be seen apart.Using the Rayleigh criterion it is possible to calculate a value for the resolving power of the eye. If the wavelength of light is 500nm and the diameter of the pupil is 5mm the using the equation θ = 1.22λ/a we can deduce that the points will not be resolved if the angle between them is less than 1.2x 10-4 radians. This means that if two objects 1mm apart will be able to be distinguished at a distance of 1/1.2x10-4 mm, approximately 8m.
Measuring the SPATIAL RESOLUTION of the Eye
To measure the SPATIAL RESOLUTION of the eye two close together objects are viewed close to the eye, the distance between the eye and the objects is then increased until they can no longer be seen as two separate lines. A commonly used variation of this is to use a series of lines and decide at what distance they appear to be continuous. Pic.5 shows an example of this. As the page is moved from the eye, at some distance x the lines appear to be the same as the filled square. If the separation of the lines is d then the resolving power is d/x radians. A trial of this with a line separation of 0.78mm revealed that the lines became indistinguishable at a distance of 185 ±2 cm this gives a SPATIAL RESOLUTION of about 4 x 10-4 rads which is not as good as that predicted by the Rayleigh criterion.
www.physicscurriculum.com
www.electron6.phys.utk.edu
www.familyconnect.com
www.wikipedia.com/angularresolution
Harten, U.: Physik für Mediziner, 11. Auflage, Springer Verlag, Heidelberg, 2006



